March 11, 2023
Hexagonal Discrete Global Grid Systems in Topographical and Hydrological Modeling

Geographic Information Systems (GIS) have dominated geospatial analysis since the 1960s, where spatial phenomena were normally represented by individual thematic layers at a specific resolution, and commonly projected to a two-dimensional Cartesian coordinate system for analysis. Discrete Global Grid Systems (DGGS), as a “congruent geography”, hierarchically partition the Earth’s surface into nearly uniform cells at various resolutions to provide great opportunities for innovation of legacy GIS (Figure 1). In the past few decades, the development of DGGS focused on fundamental implementations, such as polyhedral projections, indexing mechanisms, and generation of hierarchical grids, while limited efforts were given to the applicability studies in real-world scenarios. Our research aimed to bridge the gap between the existing DGGS implementations and DGGS-driven decision-making in the real world, and specifically, demonstrate the applicability of hexagonal DGGS in the domain of topographical and hydrological modeling.
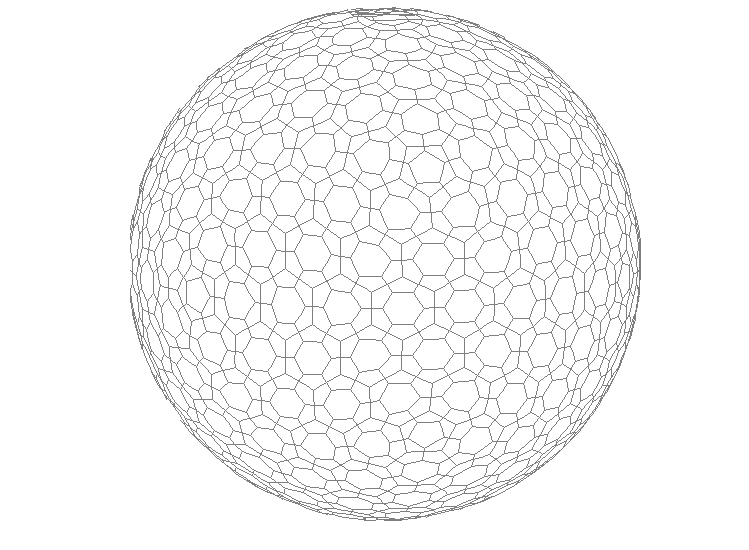
Figure 1. Icosahedral Snyder Equal Area Aperture 3 Hexagonal (ISEA3H) DGGS at two successive resolution levels.
To achieve the above goal, our research first reviewed the functional operations in a DGGS environment and compared them to those in the traditional GIS, and the outcomes were documented in the paper “Geospatial Operations of Discrete Global Grid Systems – A Comparison with Traditional GIS”. We then focused on the terrain data integration, management, and analysis in the Icosahedral Snyder Equal Area Aperture 3 Hexagonal Grid (ISEA3H) DGGS. Specifically, the integration process, including quantization and aggregation, of multi-source terrain data at various granularities was demonstrated. The full integration workflow was demonstrated in our work “Integration of Heterogeneous Terrain Data into Discrete Global Grid Systems”. Various topographical and hydrological functions, including slope and aspect, flow direction, flow accumulation, etc., were developed and experimented with various terrain types at different resolutions. We also referred to Tomlin’s theory regarding map algebra and extended it into the DGGS environment (Figure 2). Two papers were published on this topic: “Multi-resolution topographic analysis in hexagonal Discrete Global Grid Systems” and “Geovisualization of Hydrological Flow in Hexagonal Grid Systems”, focusing on topographical and hydrological operations, respectively. Finally, external machine learning algorithms were incorporated into the DGGS and used to predict future flood risks under multiple climate change scenarios, which was included in our paper “Multi-scale Flood Mapping under Climate Change Scenarios in Hexagonal Discrete Global Grids”. Figure 3 visualizes the flooding extent along with its probabilities of predicted flood risks under three climate change scenarios around our study area in the future 80 years.

Figure 2. Classification of the developed operations in this research.

Figure 3. Visualization of (a) flooding extent; and (b) probabilities of flooding predicted by the ensemble model under three climate change scenarios (RCP2.6, RCP4.5, and RCP8.5) around Fredericton, New Brunswick, in the years 2040, 2060, 2080, and 2100 at the finest modeling level.
In summary, this research promoted the adoption of DGGS in real-world decision-making, particularly in topographical and hydrological modeling. The proposed methodology for heterogenous data integration can facilitate the development of a national elevation data service in the future. The developed spatial operations can enhance the analytical functionality of hexagonal DGGS. The application in flood mapping indicated the feasibility of DGGS as the standard data fabric for multi-source data integration and multi-scale data mining.
List of publications out of this research:
1. Li, M.; Stefanakis, E. Geospatial Operations of Discrete Global Grid Systems – A Comparison with Traditional GIS. Journal of Geovisualization and Spatial Analysis, 2020, 4(2), 26.
2. Li, M.; McGrath, H.; Stefanakis, E. Integration of Heterogeneous Terrain Data into Discrete Global Grid Systems. Cartography and Geographic Information Science, 2021, 48(6), 546-564.
3. Li, M.; McGrath, H.; Stefanakis, E. Multi-resolution topographic analysis in hexagonal Discrete Global Grid Systems. International Journal of Applied Earth Observation and Geoinformation, 2022, 113, 102985.
4. Li, M.; McGrath, H.; Stefanakis, E. Geovisualization of Hydrological Flow in Hexagonal Grid Systems. Geographies, 2022, 2(2), 227-244.
5. Li, M.; McGrath, H.; Stefanakis, E. Multi-scale Flood Mapping under Climate Change Scenarios in Hexagonal Discrete Global Grids. ISPRS International Journal of GeoInformation, 2022, 11(12), 627.
